We have already discussed current, resistance, and voltage.
Now we shall discuss the important relationship that exists between the three.
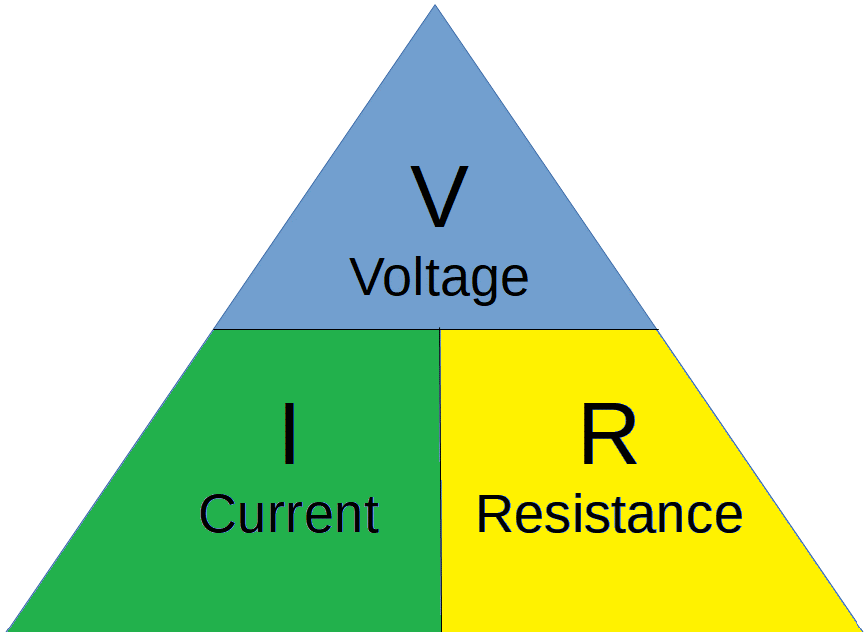
Around 1840, German physicist Georg Ohm noted that there was a distinct mathematical relationship between Voltage, current and resistance. He then wrote the basis for what we now call OHMS LAW.
Ohm’s Law states that Voltage (in Volts) is equal to the product of the current flowing through a resistance within a circuit. In other words… Voltage = Current times Resistance.
While we measure Voltage in Volts, we often use the letter E to represent Voltage. This is because another word for Voltage is ELECTROMOTIVE FORCE, which is shortened to EMF or simply E.
We use the letter I to represent the current. So that our formula becomes:

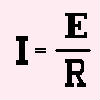

So what does this mean?
Simply put, if we have a resistance of 10 Ohms (R=10), and a current of 10 Amps(I=10), we will have a Voltage of 100 Volts, because 10*10=100 (E=100). Ohms law can also be stated two other ways. By using basic algebra, we can turn the formula around to make it say:
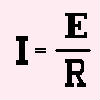 and
and 
Ohm’s Law

If we know 2 out of the 3 factors of the equation, we can figure out the third. Let’s say we know we have a 3 Volt battery. We also know we are going to put a 100 W resistor in circuit with it. How much current can we expect will flow through the circuit? Without Ohm’s Law, we would be at a loss. But because we have Ohm’s Law, we can calculate the unknown current, based upon the Voltage and Resistance.
I = 3volts/100ohms = 0.3 Amp
Let’s try solve a simple problem. We have the circuit below. We know the Voltage and the Current, because we have meters to indicate such in the circuit. When we plug in the unknown load resistance, the Voltmeter reads 45V and the Ammeter reads 2 Amperes. What is the resistance of the load?
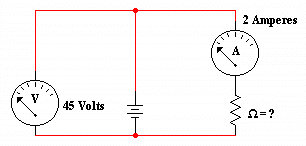
45 Volts
Resistance= ———– = 22.5 W
2 Amperes